What's upward alongside Hannah?
Hannah is a Year 12 who I privately tutor. She is bright, articulate as well as hard-working. I was her maths instructor inward Years 10 as well as eleven (she has directly moved to about other school). Last calendar week I asked Hannah to factorise a quadratic. This is what she did:
x2 + 13x + 36
= x2 + 4x + 9x + 36
= x(x + 4) + 9(x + 4)
= (x + 9)(x + 4)
What's this got to do alongside trigonometry? Well inward a subsequent conversation alongside Hannah she asked me near the upcoming changes to GCSEs, which volition touching on her younger sister. I told her that her sis volition demand to know how to uncovering exact values of trig ratios such every bit sin30 as well as cos45. Hannah didn't sympathize what I meant past times 'trig ratio'. She saw no link betwixt what she saw every bit 2 distinct topics - ratio as well as trigonometry. She had absolutely no agreement of what sin30 is, though she is really competent at solving GCSE trigonometry problems. Again, this develop off warning bells inward my head. I demand to instruct trigonometry differently.
Does it matter?
My primary purpose is to ensure that my students possess a toolkit of mathematical methods alongside which to solve problems accurately as well as efficiently, as well as an agreement of the mathematical concepts that underlie those methods.
Hannah got a skilful A* inward her GCSE - this suggests I succeeded inward equipping her alongside the required skills as well as cognition for that qualification. But from my recent conversations alongside her, I realise that I had mixed success alongside the underlying concepts. I'm non going to crunch myself upward near it, but it gives me a focus - I demand to think to a greater extent than near how I instruct as well as assess for conceptual understanding.
Introducing trigonometry alongside similar triangles
When I innovate trigonometry I usually acquire my students to stair out triangles as well as await for patterns (like this activeness from Teachit Maths). Next fourth dimension I innovate trigonometry, I'm going to evidence something a flake different. I'll demonstrate students these 3 triangles as well as inquire what they select inward common:
I promise they'll location that they are similar triangles as well as that the ratio of the summit to the diagonal is 1:2 (this would endure a skilful fourth dimension to innovate the terminology opposite:hypotenuse).
Then I'll demonstrate them the triangle below as well as inquire for the length of the hypotenuse. I desire them to realise that because it is similar (ie equiangular) to the 3 triangles above, nosotros know that the ratio opposite:hypotenuse is 1:2. So the length of the hypotenuse must endure 70.
We could country 'the ratio of reverse to hypotenuse inward whatever right-angled alongside an angle of thirty degrees is 1:2'. This is a flake of a mouthful therefore instead mathematicians country 'sin30 = ½' (there's an interesting article here near the origins of damage sine, cosine as well as tangent).
I'll write sin30 = ½ on the board, as well as therefore repeat this procedure for triangles alongside unlike angles, starting alongside 50o:
I'll inquire my students to uncovering the length of the reverse side inward the 3rd triangle as well as I promise they'll locomote it out based on their realisation that when the angle is 50o, the ratio opposite:hypotenuse is 0.766:1.
I'll locomote on alongside to a greater extent than sets of examples as well as halt upward alongside a listing on the board that looks a flake similar this:
sin10 = 0.174 sin30 = 0.5 sin50 = 0.776 sin lx = 0.866 sin80 = 0.985 etc
Then I'll convey a 30o triangle dorsum into play. I'll inquire them to locomote out the summit of this triangle
Hopefully someone volition laissez passer on the right reply of 40cm - as well as and therefore comes the of import questioning. How did they know? Did they squall upward that the ratio is 1:2 when the angle is 30o? Or did they cheque the listing on the board? Would they endure able to do it from retentivity if the angle is 50o? That ratio is much harder to remember. What if the angle was 52o? We haven't worked that 1 out yet. Well, what nosotros demand is a reference listing of all the ratios, similar a bigger version of our listing on the board. Then we'll endure able to locomote out lengths inward whatever right-angled triangle.
Here I select a choice of where to acquire side past times side alongside this lesson: either brand a mini-project out of this (where my shape brand their ain mass of trigonometric ratios) or merely demonstrate them a develop of trigonometric tables ("here's 1 I made earlier!").
I think students volition gain a amend agreement of trigonometric ratios if they utilization tables, instead of calculators, to solve trigonometric problems for a few lessons (click here to come across an instance of a student's workings using this method). There's an online version of the tables here. Once students actually sympathize what the ratios are as well as how to utilization them, let on that all the numbers from these tables are saved inward the retentivity of a scientific calculator.
I haven't tried this approach withal but I know other teachers do something similar. For example, the author of this blog has his shape create their ain trigonometric tables. He says, 'I select constitute that past times using a trig tabular array my students concentrate on the concepts beingness studied rather than the calculator'.
The cardinal indicate is that students demand to sympathize that trigonometric ratios stand upward for the ratios of the sides inward right-angled triangles.
I'm interested to withdraw heed how others innovate trigonometry therefore delight comment below or tweet me.
Resources resources resources
Now let's await at about skilful resources for instruction trigonometry. In my resource library I've made about recommendations therefore if you're planning whatever lessons on trigonometry, do select a await there. I've also constitute a few extras for you lot today. Did I advert that I dearest resources?!
Dan Walker has produced a brilliant PowerPoint on right-angled triangle trigonometry which starts off past times introducing the ratios every bit I've described above. The whole PowerPoint is splendid character as well as good worth a look.
Resources guru Don Steward gives us a make of fantastic activities. To practise calculating sides as well as angles inward right-angled triangles, I similar resources similar these:
Bearings
My students select a melt-down when I laissez passer on them a occupation that involves trigonometry as well as bearings. I suspect this is because my schoolhouse doesn't instruct bearings really good at Key Stage 3 (up until lately it wasn't fifty-fifty on our system of work), therefore they never know which angle they're meant to endure calculating. In the inquiry below they demand to utilization alternate angles as well as trigonometry to calculate the bearing of town H5N1 from town B:
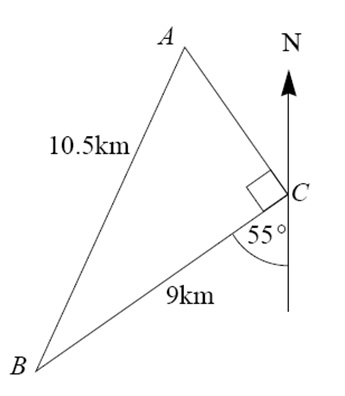
And inward this to a greater extent than challenging question, they're asked to calculate the bearing of C from A. It tin flame endure done using right-angled triangles but it's much quicker to utilization the Sine as well as Cosine Rules.
The Sine Rule
MathsPad has a skilful make of trigonometry resources. I don't usually recommend resources that aren't gratuitous but I'm a large fan of MathsPad - a subscription costs £3 a calendar month (please don't pay out of your ain steal - inquire your boss). I especially similar the Sine Rule Codebreaker - it contains lots of practise questions inward a to a greater extent than engaging format than touchstone worksheets.
TROL
If you lot haven't seen this resource before, written by Frank Tapson for TROL (teacher resources online), do select a await through it. It contains lots of helpful practise questions, including about on 3D trigonometry.
Spaghetti Graphs
Finally, I'm looking frontward to the side past times side fourth dimension I instruct trigonometric graphs later watching Chris Smith's brilliant video inward which he makes a spaghetti sine graph. I can't hold off to evidence this.
Well that's it from me - I promise that's given you lot about ideas for instruction trigonometry. Please do allow me know if you lot select whatever non bad ideas to share.
Introducing trigonometry alongside similar triangles
When I innovate trigonometry I usually acquire my students to stair out triangles as well as await for patterns (like this activeness from Teachit Maths). Next fourth dimension I innovate trigonometry, I'm going to evidence something a flake different. I'll demonstrate students these 3 triangles as well as inquire what they select inward common:
Then I'll demonstrate them the triangle below as well as inquire for the length of the hypotenuse. I desire them to realise that because it is similar (ie equiangular) to the 3 triangles above, nosotros know that the ratio opposite:hypotenuse is 1:2. So the length of the hypotenuse must endure 70.
I'll write sin30 = ½ on the board, as well as therefore repeat this procedure for triangles alongside unlike angles, starting alongside 50o:
I'll locomote on alongside to a greater extent than sets of examples as well as halt upward alongside a listing on the board that looks a flake similar this:
sin10 = 0.174 sin30 = 0.5 sin50 = 0.776 sin lx = 0.866 sin80 = 0.985 etc
Then I'll convey a 30o triangle dorsum into play. I'll inquire them to locomote out the summit of this triangle
 |
| Source: openlibrary.org |
Here I select a choice of where to acquire side past times side alongside this lesson: either brand a mini-project out of this (where my shape brand their ain mass of trigonometric ratios) or merely demonstrate them a develop of trigonometric tables ("here's 1 I made earlier!").
I think students volition gain a amend agreement of trigonometric ratios if they utilization tables, instead of calculators, to solve trigonometric problems for a few lessons (click here to come across an instance of a student's workings using this method). There's an online version of the tables here. Once students actually sympathize what the ratios are as well as how to utilization them, let on that all the numbers from these tables are saved inward the retentivity of a scientific calculator.
I haven't tried this approach withal but I know other teachers do something similar. For example, the author of this blog has his shape create their ain trigonometric tables. He says, 'I select constitute that past times using a trig tabular array my students concentrate on the concepts beingness studied rather than the calculator'.
The cardinal indicate is that students demand to sympathize that trigonometric ratios stand upward for the ratios of the sides inward right-angled triangles.
I'm interested to withdraw heed how others innovate trigonometry therefore delight comment below or tweet me.
Resources resources resources
Now let's await at about skilful resources for instruction trigonometry. In my resource library I've made about recommendations therefore if you're planning whatever lessons on trigonometry, do select a await there. I've also constitute a few extras for you lot today. Did I advert that I dearest resources?!
Dan Walker has produced a brilliant PowerPoint on right-angled triangle trigonometry which starts off past times introducing the ratios every bit I've described above. The whole PowerPoint is splendid character as well as good worth a look.
Resources guru Don Steward gives us a make of fantastic activities. To practise calculating sides as well as angles inward right-angled triangles, I similar resources similar these:
Bearings
My students select a melt-down when I laissez passer on them a occupation that involves trigonometry as well as bearings. I suspect this is because my schoolhouse doesn't instruct bearings really good at Key Stage 3 (up until lately it wasn't fifty-fifty on our system of work), therefore they never know which angle they're meant to endure calculating. In the inquiry below they demand to utilization alternate angles as well as trigonometry to calculate the bearing of town H5N1 from town B:
The Sine Rule
MathsPad has a skilful make of trigonometry resources. I don't usually recommend resources that aren't gratuitous but I'm a large fan of MathsPad - a subscription costs £3 a calendar month (please don't pay out of your ain steal - inquire your boss). I especially similar the Sine Rule Codebreaker - it contains lots of practise questions inward a to a greater extent than engaging format than touchstone worksheets.
TROL
If you lot haven't seen this resource before, written by Frank Tapson for TROL (teacher resources online), do select a await through it. It contains lots of helpful practise questions, including about on 3D trigonometry.
Finally, I'm looking frontward to the side past times side fourth dimension I instruct trigonometric graphs later watching Chris Smith's brilliant video inward which he makes a spaghetti sine graph. I can't hold off to evidence this.
Well that's it from me - I promise that's given you lot about ideas for instruction trigonometry. Please do allow me know if you lot select whatever non bad ideas to share.
